DD2365 Avancerade beräkningsmetoder i flödesmekanik 7,5 hp

• Utbildningsnivå

  Avancerad nivå

• Huvudområde

  Datalogi och datateknik
  

• Betygsskala

  A, B, C, D, E, FX, F

• Kurswebb

Lärandemål

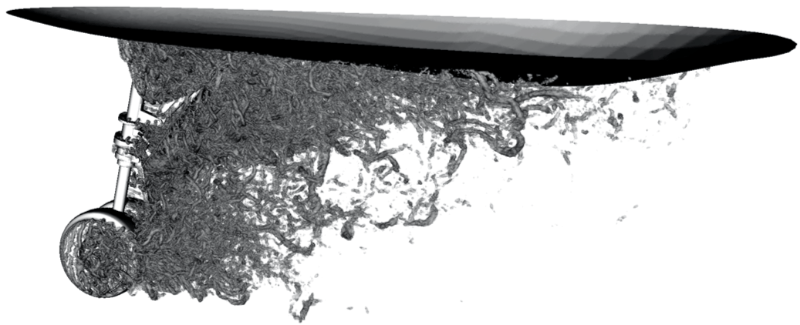
Det övergripande målet är att studenterna ska kunna analysera och använda Generell Galerkin (G2) adaptiv finita element beräkningsmetodik för att modellera strömning vid höga Reynoldstal. Konkret innebär det att studenterna ska kunna:

• redogöra för begreppen svag lösning och svag entydighet
• härleda energiuppskattningar för underliggande ekvationer samt G2 approximationer
• härleda a posteriori feluppskattningar för output i G2 med hjälp av dualitet
• analysera den globala effekten av friktionsrandvillkor i G2 beräkningar
• använda G2-programvara för adaptiva flödesberäkningar med felkontroll.

Baserat på en kritisk genomgång av forskningslitteratur samt egna beräkningsexperiment med G2, ska vidare studenterna kunna jämföra state-of-the-art strömningsmekanik med G2 beräkning/analys avseende följande fundamentala problem:

 turbulens

 separation

 generering av drag och lift

med tillämpningar inom en mängd områden som bil-, båt- och flygindustri, samt bollsporter. Avsikten är att utveckla ett eget kritiskt förhållningssätt med möjlighet att kunna ifrågasätta etablerade sanningar, samt forma egna hypoteser.

Kursens huvudsakliga innehåll

Navier-Stokes ekvationer, Eulers ekvationer, existens av exakt lösning, svag lösning, svag entydighet, Generell Galerkin (G2) metod, energiuppskattningar, perturbationstillväxt, stabilitet, dualitet, a posteriori feluppskattning och adaptivitet.

Friktionsrandvillkor, separation, gränsskikt, generering av drag och lift, Magnus-effekt, d’Alemberts paradox.

Behörighet

För fristående kursstuderande krävs 90 högskolepoäng varav 45 högskolepoäng inom matematik eller informationsteknik. Dessutom krävs engelska B eller motsvarande.

Litteratur

J. Hoffman and C. Johnson (2007) "Computational Turbulent Incompressible Flow", samt ett antal vetenskapliga artiklar (utdelas vid kursstart).

Examination

• PRO1 - Projekt, 4,0, betygsskala: P, F
• TEN1 - Tentamen, 3,5, betygsskala: A, B, C, D, E, FX, F

Krav för slutbetyg

Obligatorisk närvaro på seminarier inklusive förberedande litteraturreferat. En hemtentamen (4 hp) omfattande problem. Projektuppgift (3.5 hp).

Ges av

EECS/Datavetenskap

Kontaktperson

Johan Hoffman (jhoffman@kth.se)

Examinator

Johan Hoffman <jhoffman@kth.se>

Versionsinformation

Kursplan gäller från och med VT2019.
Examinationsinformation gäller från och med VT2019.